Complex integrals

This article concludes the topic of indefinite integrals, and includes integrals that I find quite complex. The lesson was created at the repeated requests of visitors who expressed their wish that more difficult examples be analyzed on the site.

It is assumed that the reader of this text is well prepared and knows how to apply basic integration techniques. Dummies and people who are not very confident in integrals should refer to the very first lesson - Indefinite integral. Examples of solutions, where you can master the topic almost from scratch. More experienced students can become familiar with techniques and methods of integration that have not yet been encountered in my articles.

What integrals will be considered?

First we will consider integrals with roots, for the solution of which we successively use variable replacement And integration by parts. That is, in one example two techniques are combined at once. And even more.

Then we will get acquainted with interesting and original method of reducing the integral to itself. Quite a few integrals are solved this way.

The third issue of the program will be integrals from complex fractions, which flew past the cash desk in previous articles.

Fourthly, additional integrals from trigonometric functions will be analyzed. In particular, there are methods that avoid time-consuming universal trigonometric substitution.

(2) In the integrand function, we divide the numerator by the denominator term by term.

(3) We use the linearity property of the indefinite integral. In the last integral immediately put the function under the differential sign.

(4) We take the remaining integrals. Note that in a logarithm you can use parentheses rather than a modulus, since .

(5) We carry out a reverse replacement, expressing “te” from the direct replacement:

Masochistic students can differentiate the answer and get the original integrand, as I just did. No, no, I did the check in the right sense =)

As you can see, during the solution we had to use even more than two solution methods, so to deal with such integrals you need confident integration skills and quite a bit of experience.

In practice, of course, the square root is more common; here are three examples for solving it yourself:

Example 2

Find the indefinite integral

Example 3

Find the indefinite integral

Example 4

Find the indefinite integral

These examples are of the same type, so the complete solution at the end of the article will only be for Example 2; Examples 3-4 have the same answers. Which replacement to use at the beginning of decisions, I think, is obvious. Why did I choose examples of the same type? Often found in their role. More often, perhaps, just something like .

But not always, when under the arctangent, sine, cosine, exponential and other functions there is a root of a linear function, you have to use several methods at once. In a number of cases, it is possible to “get off easy,” that is, immediately after the replacement, a simple integral is obtained, which can be easily taken. The easiest of the tasks proposed above is Example 4, in which, after replacement, a relatively simple integral is obtained.

By reducing the integral to itself

A witty and beautiful method. Let's take a look at the classics of the genre:

Example 5

Find the indefinite integral

Under the root is a quadratic binomial, and when trying to integrate this example the kettle can suffer for hours. Such an integral is taken in parts and reduced to itself. In principle, it’s not difficult. If you know how.

Let us denote the integral under consideration by a Latin letter and begin the solution:

Let's integrate by parts:

(1) Prepare the integrand function for term-by-term division.

(2) We divide the integrand function term by term. It may not be clear to everyone, but I’ll describe it in more detail:

(3) We use the linearity property of the indefinite integral.

(4) Take the last integral (“long” logarithm).

Now let's look at the very beginning of the solution:

And to the end:

What happened? As a result of our manipulations, the integral was reduced to itself!

Let's equate the beginning and the end:

Move to the left side with a change of sign:

And we move the two to the right side. As a result:

The constant, strictly speaking, should have been added earlier, but I added it at the end. I strongly recommend reading what the rigor is here:

Note: More strictly, the final stage of the solution looks like this:

Thus:

The constant can be redesignated by . Why can it be redesignated? Because he still accepts it any values, and in this sense there is no difference between constants and.
As a result:

A similar trick with constant renotation is widely used in differential equations. And there I will be strict. And here I allow such freedom only in order not to confuse you with unnecessary things and to focus attention precisely on the integration method itself.

Example 6

Find the indefinite integral

Another typical integral for independent solution. Full solution and answer at the end of the lesson. There will be a difference with the answer in the previous example!

If under the square root is quadratic trinomial, then the solution in any case comes down to two analyzed examples.

For example, consider the integral . All you need to do is first select a complete square:
.
Next, a linear replacement is carried out, which does “without any consequences”:
, resulting in the integral . Something familiar, right?

Or this example, with a quadratic binomial:
Select a complete square:
And, after linear replacement, we obtain the integral, which is also solved using the algorithm already discussed.

Let's look at two more typical examples of how to reduce an integral to itself:
– integral of the exponential multiplied by sine;
– integral of the exponential multiplied by the cosine.

In the listed integrals by parts you will have to integrate twice:

Example 7

Find the indefinite integral

The integrand is the exponential multiplied by the sine.

We integrate by parts twice and reduce the integral to itself:

As a result of double integration by parts, the integral was reduced to itself. We equate the beginning and end of the solution:

We move it to the left side with a change of sign and express our integral:

Ready. At the same time, it is advisable to comb the right side, i.e. take the exponent out of brackets, and in brackets place the sine and cosine in a “beautiful” order.

Now let's go back to the beginning of the example, or more precisely, to integration by parts:

We designated the exponent as. The question arises: is it the exponent that should always be denoted by ? Not necessarily. In fact, in the considered integral fundamentally doesn't matter, what do we mean by , we could have gone the other way:

Why is this possible? Because the exponential turns into itself (both during differentiation and integration), sine and cosine mutually turn into each other (again, both during differentiation and integration).

That is, we can also denote a trigonometric function. But, in the example considered, this is less rational, since fractions will appear. If you wish, you can try to solve this example using the second method; the answers must match.

Example 8

Find the indefinite integral

This is an example for you to solve on your own. Before you decide, think about what is more advantageous in this case to designate as , an exponential or a trigonometric function? Full solution and answer at the end of the lesson.

And, of course, do not forget that most of the answers in this lesson are quite easy to check by differentiation!

The examples considered were not the most complex. In practice, integrals are more common where the constant is both in the exponent and in the argument of the trigonometric function, for example: . Many people will get confused in such an integral, and I often get confused myself. The fact is that there is a high probability of fractions appearing in the solution, and it is very easy to lose something through carelessness. In addition, there is a high probability of an error in the signs; note that the exponent has a minus sign, and this introduces additional difficulty.

At the final stage, the result is often something like this:

Even at the end of the solution, you should be extremely careful and correctly understand the fractions:

Integrating Complex Fractions

We are slowly approaching the equator of the lesson and begin to consider integrals of fractions. Again, not all of them are super complex, it’s just that for one reason or another the examples were a little “off topic” in other articles.

Continuing the theme of roots

Example 9

Find the indefinite integral

In the denominator under the root there is a quadratic trinomial plus an “appendage” in the form of an “X” outside the root. An integral of this type can be solved using a standard substitution.

We decide:

The replacement here is simple:

Let's look at life after replacement:

(1) After substitution, we reduce the terms under the root to a common denominator.
(2) We take it out from under the root.
(3) The numerator and denominator are reduced by . At the same time, under the root, I rearranged the terms in a convenient order. With some experience, steps (1), (2) can be skipped by performing the commented actions orally.
(4) The resulting integral, as you remember from the lesson Integrating Some Fractions, is being decided complete square extraction method. Select a complete square.
(5) By integration we obtain an ordinary “long” logarithm.
(6) We carry out the reverse replacement. If initially , then back: .
(7) The final action is aimed at straightening the result: under the root we again bring the terms to a common denominator and take them out from under the root.

Example 10

Find the indefinite integral

This is an example for you to solve on your own. Here a constant is added to the lone “X”, and the replacement is almost the same:

The only thing you need to do additionally is to express the “x” from the replacement being carried out:

Full solution and answer at the end of the lesson.

Sometimes in such an integral there may be a quadratic binomial under the root, this does not change the method of solution, it will be even simpler. Feel the difference:

Example 11

Find the indefinite integral

Example 12

Find the indefinite integral

Brief solutions and answers at the end of the lesson. It should be noted that Example 11 is exactly binomial integral, the solution method of which was discussed in class Integrals of irrational functions.

Integral of an indecomposable polynomial of the 2nd degree to the power

(polynomial in denominator)

More rare, but nevertheless found in practical examples type of integral.

Example 13

Find the indefinite integral

But let’s return to the example with lucky number 13 (honestly, I didn’t guess correctly). This integral is also one of those that can be quite frustrating if you don’t know how to solve.

The solution starts with an artificial transformation:

I think everyone already understands how to divide the numerator by the denominator term by term.

The resulting integral is taken in parts:

For an integral of the form ( – natural number) withdrawn recurrent reduction formula:
, Where – integral of a degree lower.

Let us verify the validity of this formula for the solved integral.
In this case: , , we use the formula:

As you can see, the answers are the same.

Example 14

Find the indefinite integral

This is an example for you to solve on your own. The sample solution uses the above formula twice in succession.

If under the degree is indivisible square trinomial, then the solution is reduced to a binomial by isolating the perfect square, for example:

What if there is an additional polynomial in the numerator? In this case, the method of indefinite coefficients is used, and the integrand function is expanded into a sum of fractions. But in my practice there is such an example never met so I missed it this case in the article Integrals of fractional-rational functions, I'll skip it now. If you still encounter such an integral, look at the textbook - everything is simple there. I don’t think it’s advisable to include material (even simple ones), the probability of encountering which tends to zero.

Integrating complex trigonometric functions

The adjective “complicated” for most examples is again largely conditional. Let's start with tangents and cotangents in high degrees. From the point of view of the solving methods used, tangent and cotangent are almost the same thing, so I will talk more about tangent, implying that the demonstrated method for solving the integral is valid for cotangent too.

In the above lesson we looked at universal trigonometric substitution to solve a certain type of integrals from trigonometric functions. The disadvantage of universal trigonometric substitution is that its use often results in cumbersome integrals with difficult calculations. And in some cases, universal trigonometric substitution can be avoided!

Let's consider another canonical example, the integral of one divided by sine:

Example 17

Find the indefinite integral

Here you can use universal trigonometric substitution and get the answer, but there is a more rational way. I will provide the complete solution with comments for each step:

(1) We use the trigonometric formula for the sine of a double angle.
(2) We carry out an artificial transformation: Divide in the denominator and multiply by .
(3) By well-known formula in the denominator we turn the fraction into a tangent.
(4) We bring the function under the differential sign.
(5) Take the integral.

Pair simple examples for independent solution:

Example 18

Find the indefinite integral

Note: The very first step should be to use the reduction formula and carefully carry out actions similar to the previous example.

Example 19

Find the indefinite integral

Well, this is a very simple example.

Complete solutions and answers at the end of the lesson.

I think now no one will have problems with integrals:
etc.

What is the idea of the method? The idea is to use transformations and trigonometric formulas to organize only tangents and the tangent derivative into the integrand. That is, we are talking about replacing: . In Examples 17-19 we actually used this replacement, but the integrals were so simple that we got by with an equivalent action - subsuming the function under the differential sign.

Similar reasoning, as I already mentioned, can be carried out for the cotangent.

There is also a formal prerequisite for applying the above replacement:

The sum of the powers of cosine and sine is a negative integer EVEN number , For example:

for the integral – a negative integer EVEN number.

! Note : if the integrand contains ONLY a sine or ONLY a cosine, then the integral is also taken for a negative odd degree (the simplest cases are in Examples No. 17, 18).

Let's look at a couple of more meaningful tasks based on this rule:

Example 20

Find the indefinite integral

The sum of the powers of sine and cosine: 2 – 6 = –4 is a negative integer EVEN number, which means that the integral can be reduced to tangents and its derivative:

(1) Let's transform the denominator.
(2) Using the well-known formula, we obtain .
(3) Let's transform the denominator.
(4) We use the formula .
(5) We bring the function under the differential sign.
(6) We carry out replacement. More experienced students may not carry out the replacement, but it is still better to replace the tangent with one letter - there is less risk of getting confused.

Example 21

Find the indefinite integral

This is an example for you to solve on your own.

Hang in there, the championship rounds are about to begin =)

Often the integrand contains a “hodgepodge”:

Example 22

Find the indefinite integral

This integral initially contains a tangent, which immediately leads to an already familiar thought:

I will leave the artificial transformation at the very beginning and the remaining steps without comment, since everything has already been discussed above.

A couple of creative examples for your own solution:

Example 23

Find the indefinite integral

Example 24

Find the indefinite integral

Yes, in them, of course, you can lower the powers of sine and cosine, and use a universal trigonometric substitution, but the solution will be much more efficient and shorter if it is carried out through tangents. Full solution and answers at the end of the lesson

Basic trigonometric formulas and basic substitutions are presented. Methods for integrating trigonometric functions are outlined - integration rational functions, product of power functions of sin x and cos x, product of a polynomial, exponential and sine or cosine, integration of inverse trigonometric functions. Non-standard methods are affected.

Content

Standard methods for integrating trigonometric functions

General approach

First, if necessary, the integrand must be transformed so that the trigonometric functions depend on a single argument, which is the same as the integration variable.

For example, if the integrand depends on sin(x+a) And cos(x+b), then you should perform the conversion:
cos (x+b) = cos (x+a - (a-b)) = cos (x+a) cos (b-a) + sin ( x+a ) sin (b-a).
Then make the replacement z = x+a. As a result, trigonometric functions will depend only on the integration variable z.

When trigonometric functions depend on one argument that coincides with the integration variable (let's say it's z ), that is, the integrand consists only of functions like sin z, cos z, tg z, ctg z, then you need to make a substitution
.
Such a substitution leads to the integration of rational or irrational functions (if there are roots) and allows one to calculate the integral if it is integrated in elementary functions.

However, you can often find other methods that allow you to calculate the integral more the short way, based on the specifics of the integrand. Below is a summary of the main such methods.

Methods for integrating rational functions of sin x and cos x

Rational functions from sin x And cos x are functions formed from sin x, cos x and any constants using the operations of addition, subtraction, multiplication, division and raising to an integer power. They are designated as follows: R (sin x, cos x). This may also include tangents and cotangents, since they are formed by dividing sine by cosine and vice versa.
Integrals of rational functions have the form:
.

Methods for integrating rational trigonometric functions are as follows.
1) Substitution always leads to the integral of rational fraction. However, in some cases, there are substitutions (these are presented below) that lead to shorter calculations.
2) If R (sin x, cos x) cos x → - cos x sin x.
3) If R (sin x, cos x) multiplied by -1 when replacing sin x → - sin x, then the substitution t = cos x.
4) If R (sin x, cos x) does not change as with simultaneous replacement cos x → - cos x, And sin x → - sin x, then the substitution t = tg x or t = ctg x.

Examples:
, , .

Product of power functions of cos x and sin x

Integrals of the form

are integrals of rational trigonometric functions. Therefore, the methods outlined in the previous section can be applied to them. Methods based on the specifics of such integrals are discussed below.

If m and n are rational numbers, then one of the substitutions t = sin x or t = cos x the integral is reduced to the integral of the differential binomial.

If m and n are integers, then integration is performed using reduction formulas:

;
;
;
.

Example:
.

Integrals of the product of a polynomial and sine or cosine

Integrals of the form:
, ,
where P(x) is a polynomial in x, are integrated by parts. This produces the following formulas:

;
.

Examples:
, .

Integrals of the product of a polynomial, exponential and sine or cosine

Integrals of the form:
, ,
where P(x) is a polynomial in x, integrated using Euler’s formula
e iax = cos ax + isin ax(where i 2 = - 1 ).
To do this, using the method outlined in the previous paragraph, calculate the integral
.
By separating the real and imaginary parts from the result, the original integrals are obtained.

Example:
.

Non-standard methods for integrating trigonometric functions

Below are a number non-standard methods, which allow you to perform or simplify the integration of trigonometric functions.

Dependence on (a sin x + b cos x)

If the integrand depends only on a sin x + b cos x, then it is useful to apply the formula:
,
Where .

For example

Resolving fractions from sines and cosines into simpler fractions

Consider the integral
.
The simplest method of integration is to decompose the fraction into simpler ones using the transformation:
sin(a - b) = sin(x + a - (x + b)) = sin(x+a) cos(x+b) - cos(x+a) sin(x+b)

Integrating fractions of the first degree

When calculating the integral
,
it is convenient to isolate the integer part of the fraction and the derivative of the denominator
a 1 sin x + b 1 cos x = A (a sin x + b cos x) + B (a sin x + b cos x)′ .
The constants A and B are found by comparing the left and right sides.

Used literature:
N.M. Gunter, R.O. Kuzmin, Collection of problems in higher mathematics, “Lan”, 2003.

Integrating sine, cosine, tangent and cotangent

Let's start with methods for integrating the basic trigonometric functions - sin, cos, t g, c t g. Using the table of antiderivatives, we immediately write down that ∫ sin x d x = - cos x + C, and ∫ cos x d x = sin x + C.

To calculate the indefinite integrals of the functions t g and c t g, you can use the differential sign:

∫ t g x d x = ∫ sin x cos x d x = d (cos x) = - sin x d x = = - ∫ d (cos x) cos x = - ln cos x + C ∫ c t g x d x = ∫ cos x sin x d x = d (sin x) = cos x d x = = ∫ d (sin x) sin x = ln sin x + C

How did we get the formulas ∫ d x sin x = ln 1 - cos x sin x + C and ∫ d x cos x = ln 1 + sin x cos x + C, taken from the table of antiderivatives? Let us explain only one case, since the second will be clear by analogy.

Using the substitution method, we write:

∫ d x sin x = sin x = t ⇒ x = a r c sin y ⇒ d x = d t 1 - t 2 = d t t 1 - t 2

Here we need to integrate the irrational function. We use the same substitution method:

∫ d t t 1 - t 2 = 1 - t 2 = z 2 ⇒ t = 1 - z 2 ⇒ d t = - z d z 1 - z 2 = = ∫ - z d z z 1 - z 2 1 - z 2 = ∫ d z z 2 - 1 = ∫ d z (z - 1) (z +) = = 1 2 ∫ d z z - 1 - 1 2 ∫ d z z + 1 = 1 2 ln z - 1 - 1 2 z + 1 + C = = 1 2 ln z - 1 z + 1 + C = ln z - 1 z + 1 + C

Now we make the reverse substitution z = 1 - t 2 and t = sin x:

∫ d x sin x = ∫ d t t 1 - t 2 = ln z - 1 z + 1 + C = = ln 1 - t 2 - 1 1 - t 2 + 1 + C = ln 1 - sin 2 x - 1 1 - sin 2 x + 1 + C = = ln cos x - 1 cos x + 1 + C = ln (cos x - 1) 2 sin 2 x + C = = ln cos x - 1 sin x + C

We will separately analyze cases with integrals that contain powers of trigonometric functions, such as ∫ sin n x d x, ∫ cos n x d x, ∫ d x sin n x, ∫ d x cos n x.

You can read about how to calculate them correctly in the article on integration using recurrence formulas. If you know how these formulas are derived, you can easily take integrals like ∫ sin n x · cos m x d x with natural m and n.

If we have a combination of trigonometric functions with polynomials or exponential functions, then they will have to be integrated by parts. We recommend reading an article devoted to methods for finding integrals ∫ P n (x) · sin (a x) d x , ∫ P n (x) · cos (a x) d x , ∫ e a · x · sin (a x) d x , ∫ e a · x · cos (a x) d x .

The most difficult problems are those in which the integrand includes trigonometric functions with different arguments. To do this, you need to use basic trigonometry formulas, so it is advisable to memorize them or keep a note of them at hand.

Example 1

Find the set of antiderivatives of the function y = sin (4 x) + 2 cos 2 (2 x) sin x · cos (3 x) + 2 cos 2 x 2 - 1 · sin (3 x) .

Solution

Let's use the formulas for reducing the degree and write that cos 2 x 2 = 1 + cos x 2, and cos 2 2 x = 1 + cos 4 x 2. Means,

y = sin (4 x) + 2 cos 2 (2 x) sin x cos (3 x) + 2 cos 2 x 2 - 1 sin (3 x) = sin (4 x) + 2 1 + cos 4 x 2 sin x · cos (3 x) + 2 · 1 + cos x 2 - 1 · sin (3 x) = = sin (4 x) + cos (4 x) + 1 sin x · cos (3 x) + cos x sin (3 x)

In the denominator we have the formula for the sine of the sum. Then you can write it like this:

y = sin (4 x) + cos (4 x) + 1 sin x cos (3 x) + cos x sin (3 x) = sin (4 x) + cos (4 x) + 1 sin (4 x ) = = 1 + cos (4 x) sin (4 x)

We got the sum of 3 integrals.

∫ sin (4 x) + cos (4 x) + 1 sin x · cos (3 x) + cos x · sin (3 x) d x = = ∫ d x + cos (4 x) d x sin (4 x) + ∫ d x sin (4 x) = = x + 1 4 ln ∫ d (sin (4 x)) sin (4 x) + 1 4 ln cos (4 x) - 1 sin (4 x) = = 1 4 ln sin ( 4 x) + 1 4 ln cos (4 x) - 1 sin (4 x) + C = x + 1 4 ln cos 4 x - 1 + C

In some cases, trigonometric functions under the integral can be reduced to fractional rational expressions using the standard substitution method. First, let's take formulas that express sin, cos and t g through the tangent of the half argument:

sin x = 2 t g x 2 1 + t g 2 x 2 , sin x = 1 - t g 2 x 2 1 + t g 2 x 2 , t g x = 2 t g x 2 1 - t g 2 x 2

We will also need to express the differential d x in terms of the tangent of the half angle:

Since d t g x 2 = t g x 2 "d x = d x 2 cos 2 x 2, then

d x = 2 cos 2 x 2 d t g x 2 = 2 d t g x 2 1 cos 2 x 2 = 2 d t g x 2 cos 2 x 2 + sin 2 x 2 cos 2 x 2 = 2 d t g x 2 1 + t g 2 x 2

Thus, sin x = 2 z 1 + z 2, cos x 1 - z 2 1 + z 2, t g x 2 z 1 - z 2, d x = 2 d z 1 + z 2 at z = t g x 2.

Example 2

Find the indefinite integral ∫ d x 2 sin x + cos x + 2 .

Solution

We use the standard trigonometric substitution method.

2 sin x + cos x + 2 = 2 2 z 1 + z 2 + 1 - z 2 1 + z 2 = z 2 + 4 z + 3 1 + z 2 ⇒ d x 2 sin x + cos x + 2 = 2 d z 1 + z 2 z 2 + 4 z + 3 1 + z 2 = 2 d z z 2 + 4 z + 3

We obtain that ∫ d x 2 sin x + cos x + 2 = 2 d z z 2 + 4 z + 3 .

Now we can expand the integrand into simple fractions and obtain the sum of two integrals:

∫ d x 2 sin x + cos x + 2 = 2 ∫ 2 d z z 2 + 4 z + 3 = 2 ∫ 1 2 1 z + 1 - 1 z + 3 d z = = ∫ d z z + 1 - ∫ C z + 3 = ln z + 1 - ln z + 3 + C = ln z + 1 z + 3 + C

∫ d x 2 sin x + cos x + 2 = ln z + 1 z + 3 + C = ln t g x 2 + 1 t g x 2 + 3 + C

Answer: ∫ d x 2 sin x + cos x + 2 = ln t g x 2 + 1 t g x 2 + 3 + C

It is important to note that those formulas that express functions through the tangent of a half argument are not identities, therefore, the resulting expression ln t g x 2 + 1 t g x 2 + 3 + C is the set of antiderivatives of the function y = 1 2 sin x + cos x + 2 only on the domain of definition.

To solve other types of problems, you can use basic integration methods.

If you notice an error in the text, please highlight it and press Ctrl+Enter

There will also be problems for you to solve on your own, to which you can see the answers.

The integrand can be converted from the product of trigonometric functions to the sum

Let us consider integrals in which the integrand is the product of sines and cosines of the first degree of x multiplied by different factors, that is, integrals of the form

Using well-known trigonometric formulas

(2)
(3)
(4)
one can transform each of the products in integrals of the form (31) into an algebraic sum and integrate according to the formulas

(5)

(6)

Example 1. Find

Solution. According to formula (2) at

Example 2. Find integral of a trigonometric function

Solution. According to formula (3) at

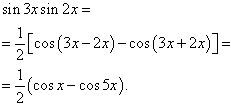
Example 3. Find integral of a trigonometric function

Solution. According to formula (4) at we obtain the following transformation of the integrand:

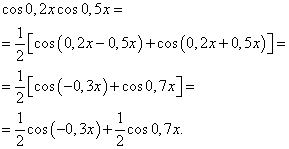
Applying formula (6), we obtain

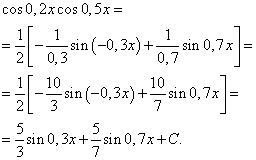
Integral of the product of powers of sine and cosine of the same argument

Let us now consider integrals of functions that are the product of powers of sine and cosine of the same argument, i.e.

(7)

In special cases, one of the indicators ( m or n) may be zero.

When integrating such functions, it is used that an even power of cosine can be expressed through sine, and the differential of sine is equal to cos x dx(or even power of sine can be expressed in terms of cosine, and the differential of cosine is equal to - sin x dx ) .

Two cases should be distinguished: 1) at least one of the indicators m And n odd; 2) both indicators are even.

Let the first case take place, namely the indicator n = 2k+ 1 - odd. Then, given that

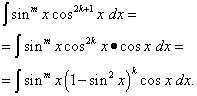
The integrand is presented in such a way that one part of it is a function of only the sine, and the other is the differential of the sine. Now using variable replacement t= sin x the solution reduces to integrating the polynomial with respect to t. If only the degree m is odd, then they do the same, isolating the factor sin x, expressing the rest of the integrand in terms of cos x and believing t=cos x. This technique can also be used when integrating the quotient powers of sine and cosine , When at least one of the indicators is odd . The whole point is that the quotient of the powers of sine and cosine is special case their works : When a trigonometric function is in the denominator of an integrand, its degree is negative. But there are also cases of partial trigonometric functions, when their powers are only even. About them - in the next paragraph.

If both indicators m And n– even, then, using trigonometric formulas

lower the exponents of the sine and cosine, after which you get an integral of the same type as above. Therefore, integration should be continued according to the same scheme. If one of the even exponents is negative, that is, the quotient of even powers of sine and cosine is considered, then this scheme no good . Then a change of variable is used depending on how the integrand can be transformed. Such a case will be considered in the next paragraph.

Example 4. Find integral of a trigonometric function

Solution. The cosine exponent is odd. Therefore, let's imagine

t= sin x(Then dt=cos x dx ). Then we get

Returning to the old variable, we finally find

Example 5. Find integral of a trigonometric function

Solution. The cosine exponent, as in the previous example, is odd, but larger. Let's imagine

and make a change of variable t= sin x(Then dt=cos x dx ). Then we get

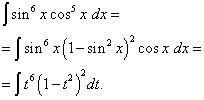
Let's open the brackets

and we get

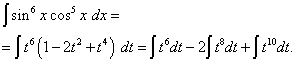
Returning to the old variable, we get the solution

Example 6. Find integral of a trigonometric function

Solution. The exponents of sine and cosine are even. Therefore, we transform the integrand function as follows:

Then we get

In the second integral we make a change of variable, setting t= sin2 x. Then (1/2)dt= cos2 x dx . Hence,

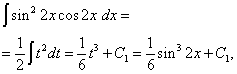
Finally we get

Using the Variable Replacement Method

Variable Replacement Method when integrating trigonometric functions, it can be used in cases where the integrand contains only sine or only cosine, the product of sine and cosine, in which either sine or cosine is to the first degree, tangent or cotangent, as well as the quotient of even powers of sine and cosine of one and the same argument. In this case, it is possible to perform permutations not only sin x = t and sin x = t, but also tg x = t and ctg x = t .

Example 8. Find integral of a trigonometric function

Solution. Let's change the variable: , then . The resulting integrand can be easily integrated using the table of integrals:

Example 9. Find integral of a trigonometric function

Solution. Let's transform the tangent into the ratio of sine and cosine:

Let's change the variable: , then . The resulting integrand is table integral with a minus sign:

Returning to the original variable, we finally get:

Example 10. Find integral of a trigonometric function

Solution. Let's change the variable: , then .

Let's transform the integrand to apply the trigonometric identity :

We change the variable, not forgetting to put a minus sign in front of the integral (see above, what is equal to dt). Next, we factor the integrand and integrate according to the table:

Returning to the original variable, we finally get:

Find the integral of a trigonometric function yourself, and then look at the solution

Universal trigonometric substitution

Universal trigonometric substitution can be used in cases where the integrand does not fall under the cases discussed in the previous paragraphs. Basically, when sine or cosine (or both) is in the denominator of a fraction. It has been proven that sine and cosine can be replaced by another expression containing the tangent of half the original angle as follows:

But note that universal trigonometric substitution often entails quite complex algebraic transformations, so it is best used when no other method will work. Let us look at examples where, together with the universal trigonometric substitution, subsumption under the differential sign and the method of indefinite coefficients are used.

Example 12. Find integral of a trigonometric function

Solution. Solution. Let's take advantage universal trigonometric substitution. Then
.

We multiply the fractions in the numerator and denominator by , and take out the two and place it in front of the integral sign. Then